DAMQT: Overview: An illustrative example

An illustrative example

In a LCAO calculation of H₂ carried out with a minimal basis set, the density results:

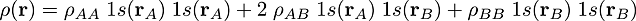
(1)

where

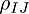are the elements of the density matrix,

,

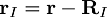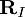stands for the position of the nuclei

(

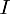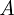or

), and

is either a

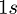STO or the corresponding combination of Gaussian primitives. The first and third terms in (1) contain the spherical charge distributions,

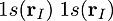centered at the nuclei

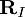. The second term has the two-center charge distribution,

extending along the internuclear axis.
Depicting the values of these distributions along the internuclear axis one has:

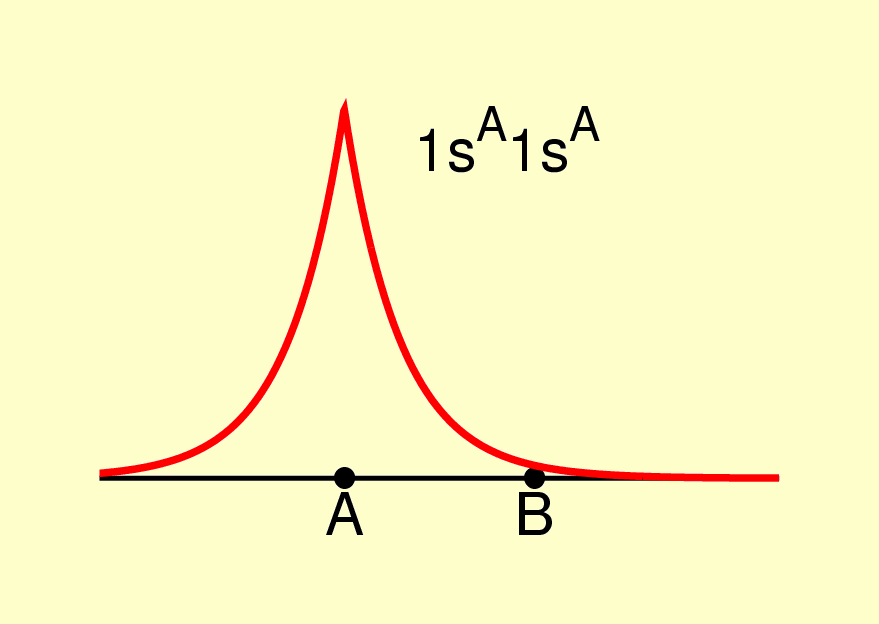
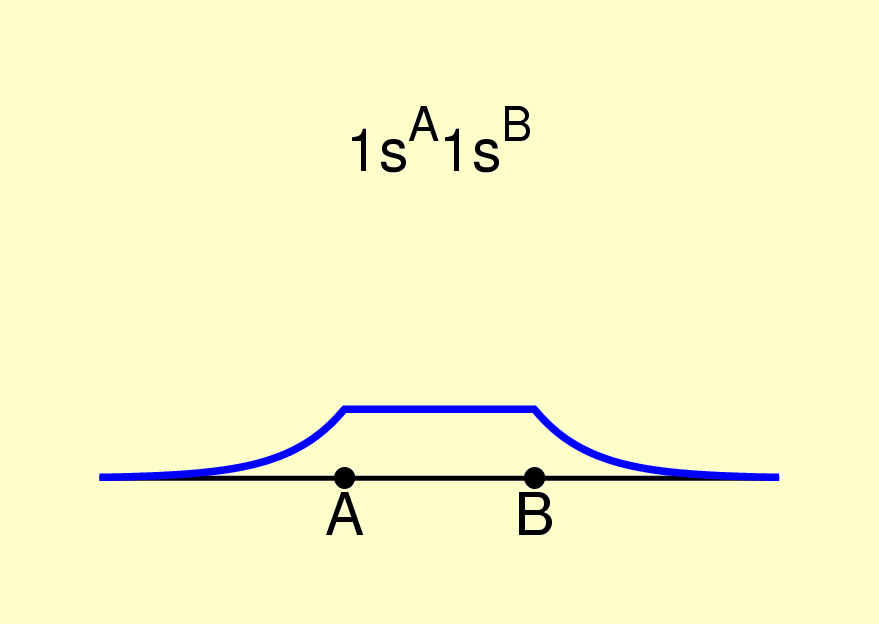
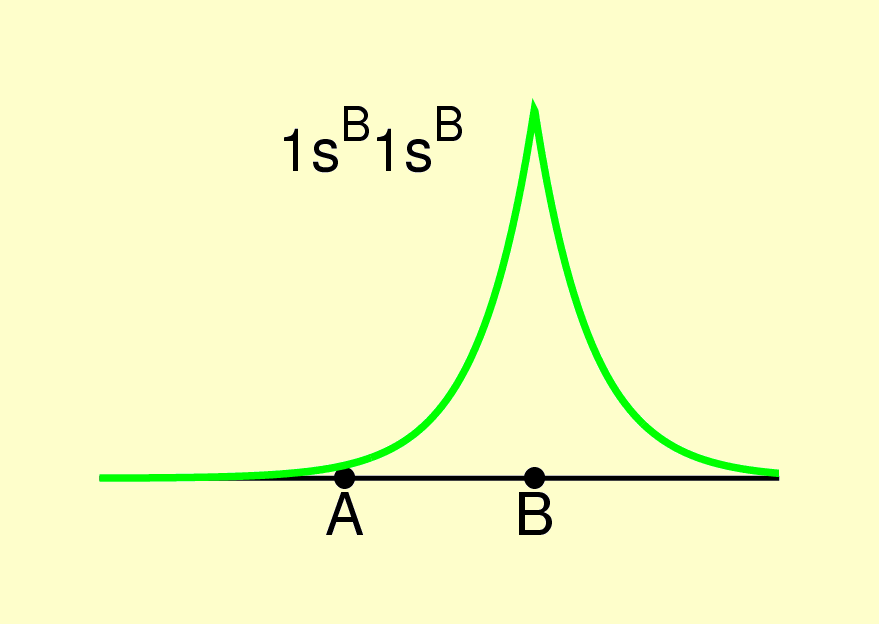
The one-center contributions to the density are:

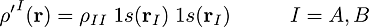
(2)

The two-center contributions are obtained after partitioning the two-center distribution into two minimally deformed fagments:

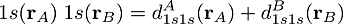
(3)

This partitioning is illustrated in the next figure, where the full distribution,

and the

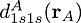and

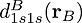fragments have been plotted along the internuclear axis: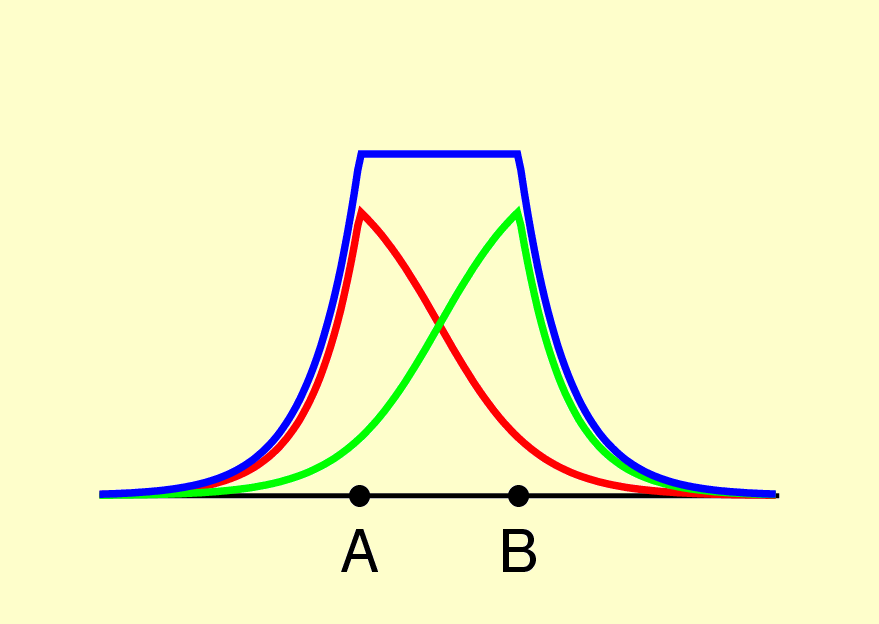

The two-center contributions are:

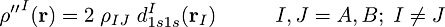
(4)

The

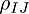elements of the density matrix can be obtained with different methods. Taking

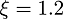and

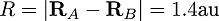, and employing the VB, RHF and CI methods for

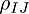one obtains the following pictures of

,

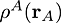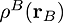, and

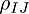along the internuclear axis:

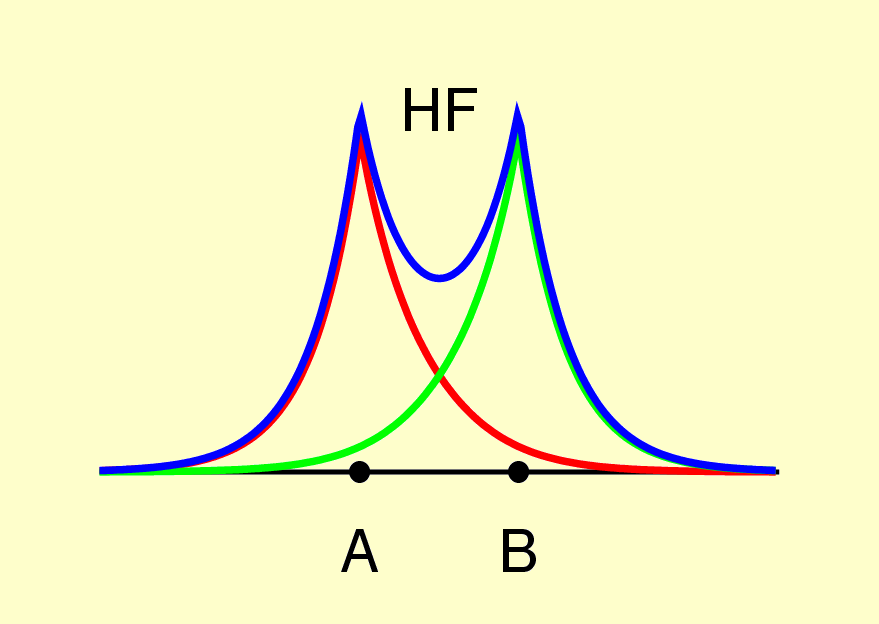
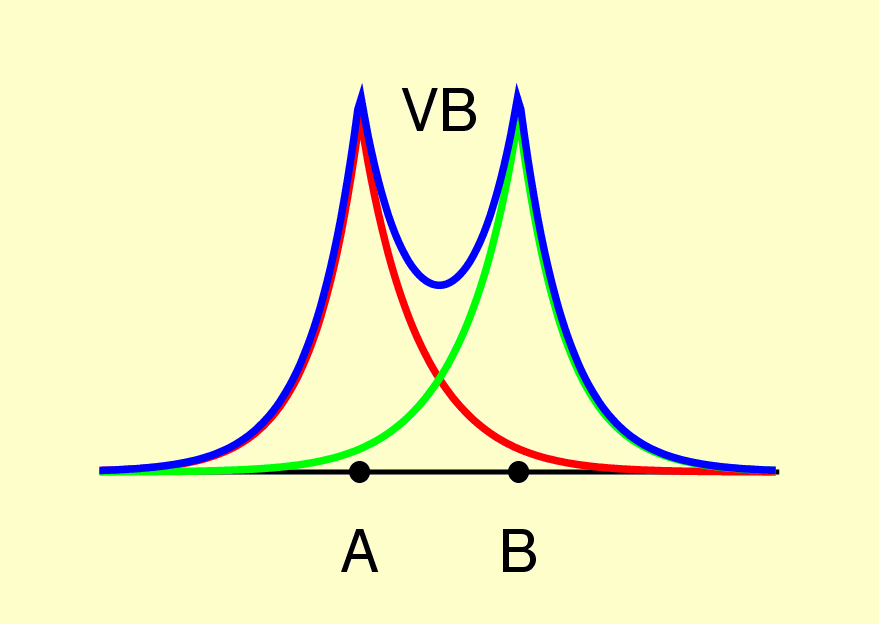
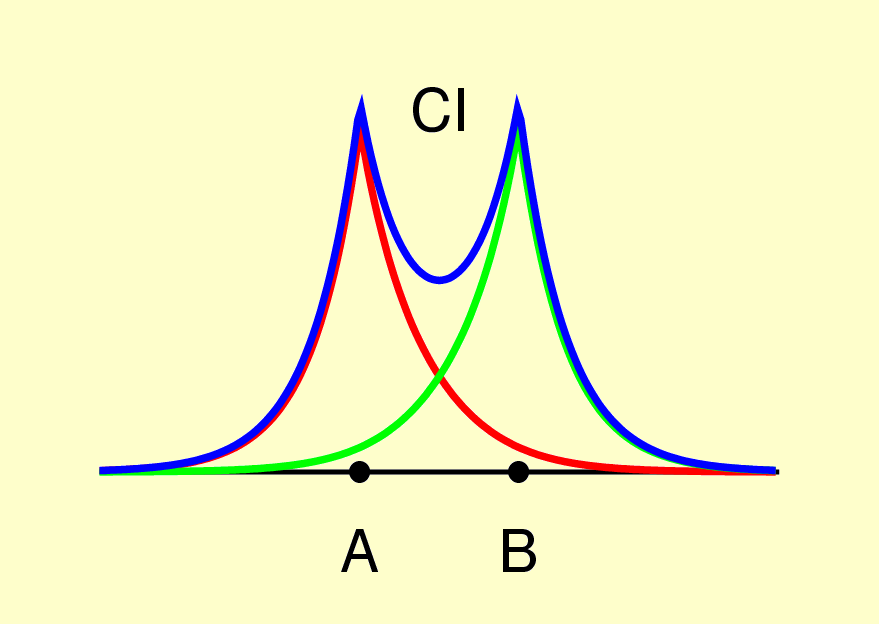
The last step in the method is the expansion of

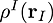in spherical harmonics centered at

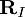times radial factors: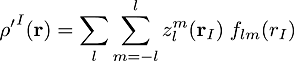

(5)

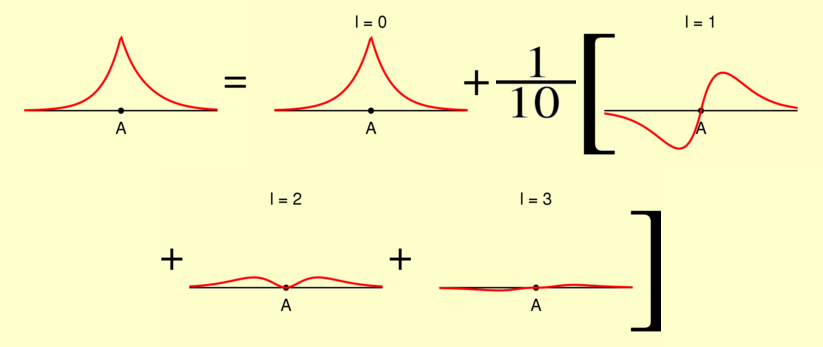
This expansion is illustrated in the following figures by drawing the values of the first terms along the internuclear axis. The terms with

have been multiplied by 10 for clarity.